For any given line R and point P not on R in the plane containing both line R and point P there are at least two distinct lines through P that do not intersect R. Accordingly this book introduces the notion of vectors into analytic hyperbolic.

The Gyrotriangle And Its Standard Notation In An Einstein Gyrovector Download Scientific Diagram
The essay has been lightly edited before being published here.
. Hyperbolic line segments are congruent if they have the same length. The premise of analogy as a study strategy is to make the unfamiliar familiar. It introduces the notion of vectors into analytic hyperbolic geometry where they are called gyrovectors.
It introduces the notion of vectors into analytic hyperbolic geometry where they are called gyrovectorsNewtonian velocity addition is the common vector addition which is both. In mathematics hyperbolic geometry also called Lobachevskian geometry or BolyaiLobachevskian geometry is a non-Euclidean geometryThe parallel postulate of Euclidean geometry is replaced with. This book presents a powerful way to study Einsteins special theory of relativity and its underlying hyperbolic geometry in which analogies with classical results form the right tool.
If a proof in Euclidean geometry could be found that proved the parallel. The image that Escher presents is a distorted map of the hyperbolic world. Up to 10 cash back The relativistically admissible velocities of Einsteins special theory of relativity are regulated by the BeltramiKlein ball model of the hyperbolic geometry of Bolyai and Lobachevsky.
Following a brief review of the history of the link between Einsteins velocity addition law of special relativity and the hyperbolic geometry of Bolyai and Lobachevski we employ the binary operation of Einsteins velocity addition to introduce into hyperbolic geometry the concepts of vectors angles and trigonometry. The discovery of Vladimir Variak in 1908-1910 910 that Einsteins addition of relativistically admissible three-velocities has natural interpretation in the hyperbolic geometry of Bolyai and Lobachevsky was therefore a great triumph to Riemann and to the principle of harmony between mathematics and physics. This essay is an introduction to the history of hyperbolic geometry.
History of Hyperbolic Geometry. Analytic Hyperbolic Geometry And Albert Einsteins Special Theory Of Relativity. A It makes possible the reformulation of Einsteins Doppler shift law in a way completely.
The points A B B0 are any three points in an Einstein gyrovector space Vs that do not lie on a geodesic line and the point A0is given by the hyperbolic parallelogram condition 41. The hyperbolic distance between points P Q in the Poincare disk is dP Q cosh 1 1 2jPQj2 1 jPj21 jQj2. A flat or Euclidean plane has zero curvature.
Geometry where they are called gyrovectors the use of analytic hyperbolic geometry extends Einsteins unfinished symphony significantly elevating it. It is shown in this expository article that the Einstein velocity addition law of relativistically admissible velocities enables Cartesian coordinates to. To understand how Einsteins ideas came to influence geome try.
Later physicists discovered practical applications of these ideas to the theory of. Euclid Gauss Felix Klein and Henri Poincare all made major. It was Riemannian geometry that enabled Einstein to establish a physical bridge between light matter and space-time and translate his.
Sami was a student in the Fall 2016 course Geometry of Surfaces taught by Scott Taylor at Colby College. As we all know Einsteins monumental contribution was the replacement of the Newtonian theory of gravity by what is called General Relativity. The hyperbolic h geometry of relativity represents the velocity addition law as a triangle on the surface of a pseudosphere a surface of revolution looking like a bugle and the angle of parallelism which measures the deviation from Euclidean e space.
The equations were first published by Einstein in 1915 in the form of a tensor equation which related the local spacetime curvature expressed by the Einstein tensor with the local energy momentum and. This book presents a powerful way to study Einsteins special theory of relativity and its underlying hyperbolic geometry in which analogies with classical results form the right tool. Also known as Einsteins equations relate the geometry of spacetime to the distribution of matter within it.
In Eschers work hyperbolic space is a distorted disk. Where jPQjis the Euclidean distance and jPjjQjare the Euclidean distances of P Q from the origin. As the relative velocity approaches unity the angle of parallelism approaches zero.
This theory has two essential features the first is to move from 3-dimensional geometry to 4-dimensional geometry by incorporating. These ideas were fundamental to Einsteins special theory of relativity. The angle between hyperbolic rays is that between their Euclidean.
Newtonian velocity addition is the common vector. This book presents a powerful way to study Einsteins special theory of relativity and its underlying hyperbolic geometry in which analogies with classical results form the right tool. The Einstein Hyperbolic Parallelogram and the Relativistic Velocity Hyperbolic Parallelogram Addition Law 42.
In the general theory of relativity the Einstein field equations EFE. 152 A little algebra We will be working with special conics and quadratic curves and. We will describe hyperbolic space in several different ways.
Hyperbolic geometry is a type of non-Euclidean geometry that arose historically when mathematicians tried to simplify the axioms of Euclidean geometry and instead discovered unexpectedly that changing one of the axioms to its negation actually produced a consistent theory. The resulting gyrovector spaces in turn form the algebraic setting for the Beltrami-Klein ball model of the. This brings up the subject of hyperbolic geometry.
In the hyperbolic plane the parallel postulate is false. All of the angels in Circle Limit IV Heaven and Hell live in hyperbolic space where they are actually the same size as do the devil figures. The use of hyperbolic velocity leads to a simplification of several laws in special relativity.
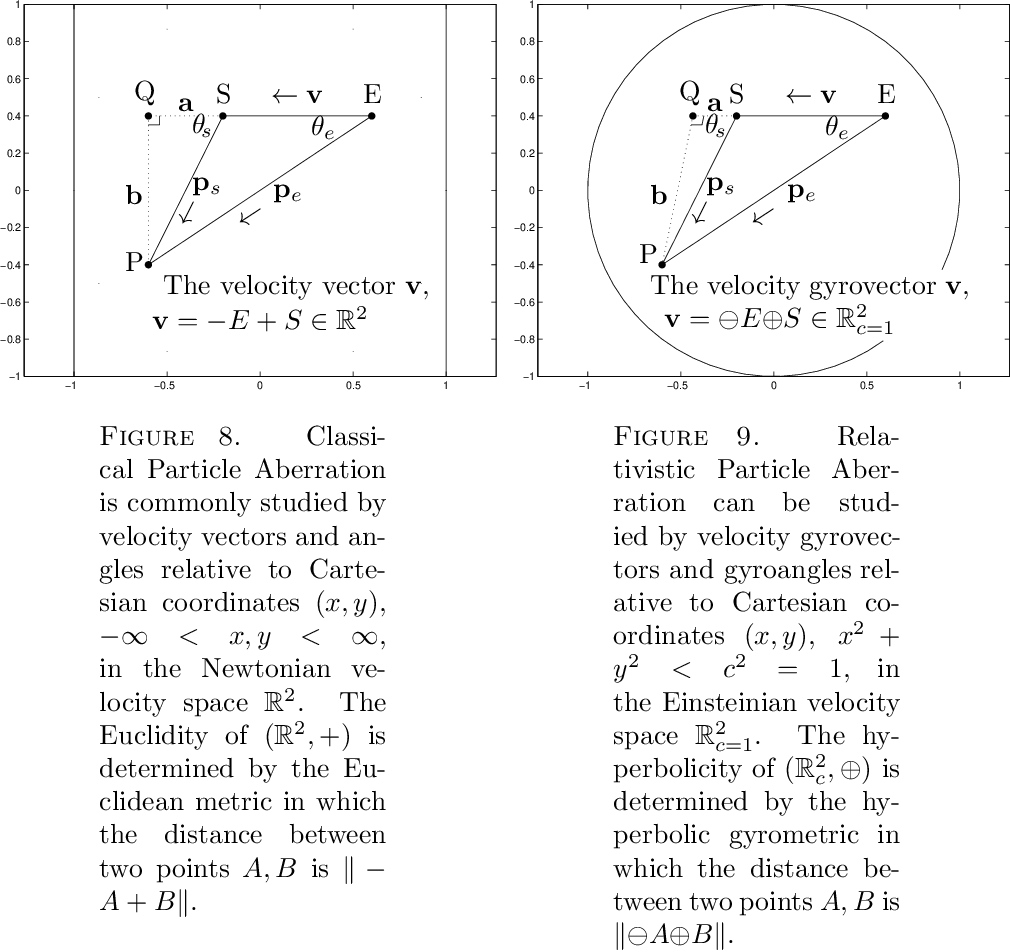
In full analogy Einsteinian velocity addition is a gyrovector addition which is both gyrocommutative and gyroassociative. The surface of a sphere like a beach ball has positive curvature and a hyperbolic plane has negative curvature.

Pdf Einstein S Special Relativity The Hyperbolic Geometric Viewpoint Semantic Scholar

Pdf Einstein S Special Relativity The Hyperbolic Geometric Viewpoint Semantic Scholar

Pdf Einstein S Special Relativity The Hyperbolic Geometric Viewpoint Semantic Scholar
0 Comments